Clipart tagged: ‘spirals’
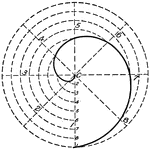
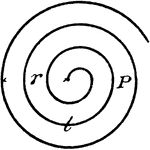
Draw Spiral of Archimedes
"Divide the circumference into a number of equal parts, drawing the radii and numbering the points.…
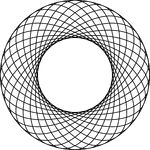
16 Rotated Concentric Ellipses
Illustration of 16 concentric congruent ellipses that are rotated about the center at equal intervals…
2 Rotated Concentric Ellipses
Illustration of 2 concentric congruent ellipses that are rotated about the center at 90°. The ellipses…
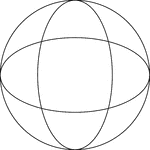
4 Rotated Concentric Ellipses
Illustration of 4 concentric congruent ellipses that are rotated about the center at equal intervals…
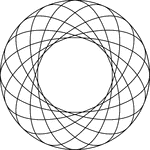
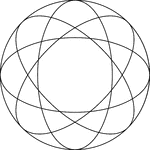
8 Rotated Concentric Ellipses
Illustration of 8 concentric congruent ellipses that are rotated about the center at equal intervals…
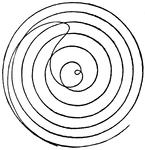
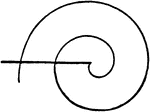
Fermat's Spiral
Also called a parabolic spiral, it is a type of Archimedean Spiral. A spiral is defined as "a plane…
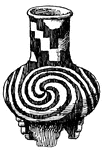
Mexican Jar with Spirals
Mexican jar with spiral design sketched in the American Museum of Natural History in New York.
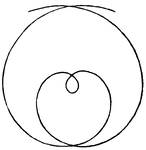
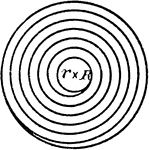
Archimedean Spiral
Illustration of a spiral named after the 3rd century BC Greek mathematician Archimedes.
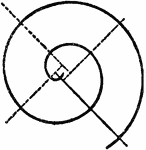
Construction Of A Spiral
An illustration showing how to construct a spiral with compasses and four centers. "Given the pitch…
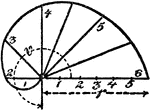
Construction Of An Arithmetic Spiral
An illustration showing how to construct an arithmetic spiral. "Given the pitch p and angle v, divide…
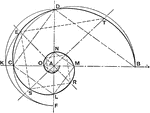
Drawing Equiangular or Logarithmic Spiral
"If B and C are points in the spiral and the ratio of AC to AB be given, the intermediate point D may…
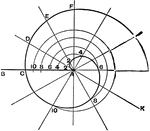
Equiangular or Logarithmic Spiral
A graph showing a logarithmic or equiangular spiral. The spiral is created in polar coordinates (r,Θ)…
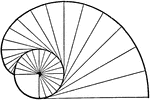
Helix Spiral
A helix spiral generated by revolving about an axis at an angle less than 90 degrees. This generates…
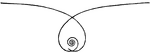
Hyperbolic Spiral
Illustration of a hyperbolic spiral, also called a reciprocal spiral, it is a transcendental plane curve.…
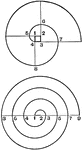
Involute Spiral
"This class of spirals may be generated by unwinding a perfectly flexible but inextensible chord from…
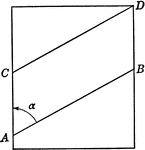
Spiral Drawn on Piece of Paper Shown Flat
Spiral with lines AB and CD drawn on piece of paper shown flat.

Spiral Drawn on Piece of Paper and Rolled
Spiral with lines AB and CD drawn on piece of paper and rolled into a cylinder to form spiral running…