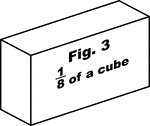
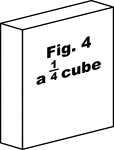
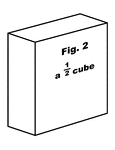
Cubes
The Cubes ClipArt gallery offers 27 images of 3-dimensional geometric solids bounded by six equal squares, making the height, width, and length equal, and all interior angles 90 degrees. It is also called a regular hexahedron, and is one of the five Platonic solids.
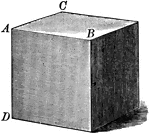
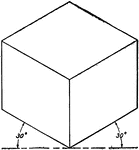
Cube
A regular body with six square faces; a rectangular parallelopiped, having all its edges equal.
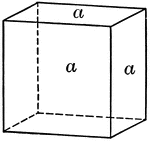
Cube
"Science has succeeded in classifying the thousands of known crystals in six systems, to each of which…
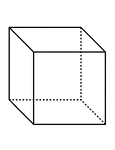
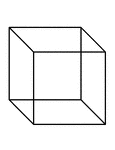
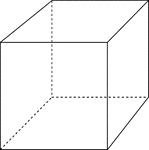
Cube
"A cube is a prism whose faces are ends are squares. All the faces of a cube are equal." —Hallock…
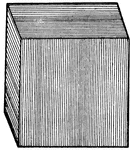
Cube of Iron Pyrite
Cubes of Iron Pyrites frequently show a striation of their planes in one direction, which is perpendicular…
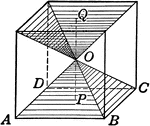
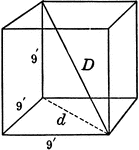
Cube for Illustrating Volume
An illustration of a cube divided into 6 equal pyramids to illustrate how volume can be found.
Cubing
A visual representation of cubing. "In the diagrams, Figure 1 represents 40 cubed and has a content…

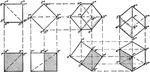
Froebel's Divided Cube (Complex)
This image shows one of Friedrich Froebel's divided cube (this one divided into many smaller cubes and…
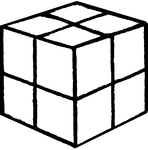
Froebel's Divided Cube (Eight Smaller Cubes)
This image shows one of Friedrich Froebel's divided cube (this one divided into eight smaller cubes).…
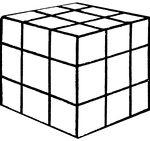
Froebel's Divided Cube (Twenty-seven Smaller Cubes)
This image shows one of Friedrich Froebel's divided cube (this one divided into twenty-seven smaller…
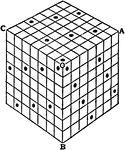
Nasik Cube
Squares that have many more summations than just rows, columns, and diagonals. Frost extended this idea…
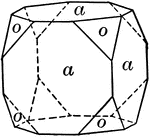
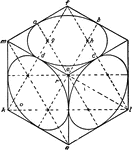
Cube trunctuated by octahedron
"When a corner or an edge of one form is replaced by a face of another form, the first is said to be…