Conic Sections: Parabolas
This mathematics ClipArt gallery offers 26 images of conic sections, or conics, creating parabolas. Conics are obtained by taking a cone, or conical surface, and intersecting it with a plane. Conic parabolas are retrieved by intersecting the cones with a plane that is parallel to the lines used to create the cone. In other words, the plane that cuts the conical surface is at the same angle as the lines that were used to make the cones, resulting in an unbounded (meaning not a closed shape)" curve that is a parabola.
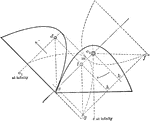
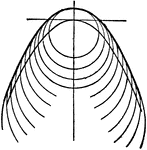
Rolling of Equal Parabolas
Illustration of the rolling of equal parabolas. "The two parabolas may be considered as two ellipses…
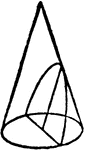
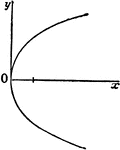
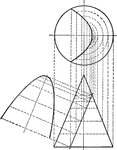
Parabola
Illustration showing a parabola as a curve formed by the intersection of the surface of a cone with…
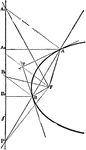
Parabola Foci Properties
The segments between the point of intersection of two tangents to a conic and their points of contact…
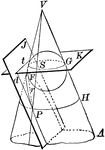
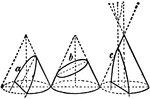
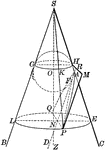
Cone Intersected by a Plane to Form a Parabola
Diagram of a cone intersected by plane J to form a parabola. Also pictured is a circle formed by the…
Conic Section Parabola
Illustration of a cone cut by a plane that is parallel to one of the elements of the cone. The curve…

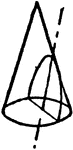
Conic Section Showing Parabola
Illustration showing the definition of a parabola as a conic section. "The section of a right circular…
Conic Section Showing Parabola
Illustration showing the definition of a parabola as a conic section. "The section of a right circular…
Conic Section Showing Parabola
Illustration showing the definition of a parabola as a conic section. The plane cuts the cone parallel…

Conic Section Showing A Parabola
Illustration showing the definition of a parabola as a conic section. The section of a right circular…
Conic Section Showing A Parabola
An illustration showing the intersection of a plane and a cone. The cone is intersected by a plane parallel…
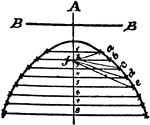
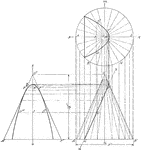
Construction Of A Parabola
An illustration showing how to construct a parabola by plotting. "Having given the axis, vertex, and…
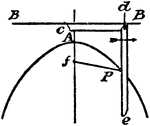
Construction Of A Parabola
An illustration showing how to construct a parabola using a pencil and a string. "Having given the two…
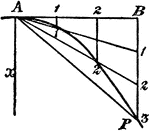
Construction Of A Parabola
An illustration showing how to construct a parabola. "Given the vertex A, axis x, and a point P. Draw…
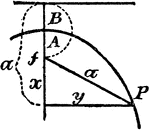
Construction Of A Parabola
An illustration showing how to construct a parabola. "Given the axis of ordinate B, and vertex A. Take…
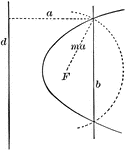
Construction of a Parabola
Diagram showing how to construct a parabola when given the directrix (d), the focus (F) and the eccentricity…
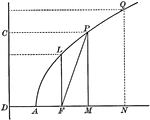
Parabola With Coordinates and Latus Rectum
Illustration of a parabola showing that any point of a parabola is the mean proportional between the…
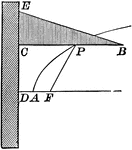
Parabola With Focus and Directrix
Illustration of a parabola - a curve which is the locus of a point that moves in a plane so that its…
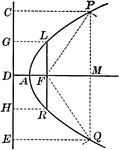
Parabola With Focus and Directrix
Illustration of a parabola - a curve which is the locus of a point that moves in a plane so that its…
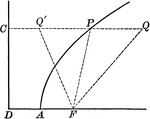
Point on Parabola
Illustration of a parabola showing point P is equidistant to the focus and the directrix.
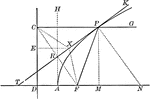
Point on Parabola
Illustration of a parabola. "If a line PT is drawn from any point P of the curve, bisecting the angle…
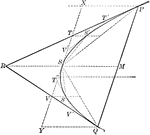
Tangents and Chords of a Parabola
Illustration showing that the area of a parabolic segment made by a chord is two thirds the area of…
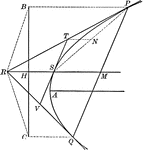
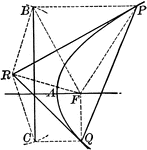
Tangents to a Parabola
Illustration showing that if two tangents RP and RQ are drawn from a point R to a parabola, the line…