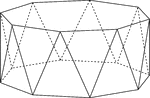
Nonagonal Antiprism
Illustration of a nonagonal antiprism. An antiprism is formed by having two parallel congruent bases…
Octagonal Antiprism
Illustration of an octagonal antiprism. An antiprism is formed by having two parallel congruent bases…
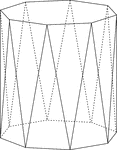
Octagonal Antiprism
Illustration of an octagonal antiprism. An antiprism is formed by having two parallel congruent bases…
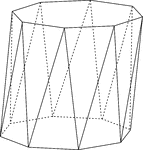
Skewed Octagonal Antiprism
Illustration of a skewed (non-right) octagonal antiprism. An antiprism is formed by having two parallel…
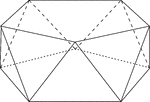
Pentagonal Antiprism
Illustration of a pentagonal antiprism. An antiprism is formed by having two parallel congruent bases…
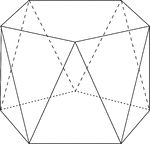
Pentagonal Antiprism
Illustration of a pentagonal antiprism. An antiprism is formed by having two parallel congruent bases…
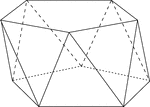
Skewed Pentagonal Antiprism
Illustration of a skewed (non-right) pentagonal antiprism. An antiprism is formed by having two parallel…
Euclid's Pythagorean Theorem Proof
Illustration used to prove the Pythagorean Theorem, according to Euclid. A perpendicular is drawn from…
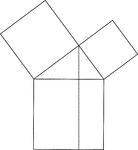
Geometric Pythagorean Theorem Proof
Illustration that can be used to prove the Pythagorean Theorem, the sum of the squares of the legs is…
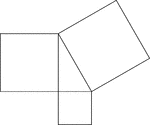
Geometric Pythagorean Theorem Proof
Illustration that can be used to prove the Pythagorean Theorem, the sum of the squares of the legs is…
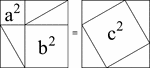
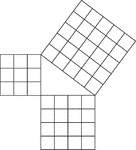
Pythagorean Theorem Proof by Rearrangement
A visual illustration used to prove the Pythagorean Theorem by rearrangement. When the 4 identical triangles…
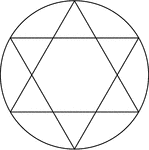
Star Inscribed In A Circle
Illustration of a 6-point star created by two equilateral triangles (often described as the Star of…

Triangle With Circle Constructions
Illustration of a triangle with its incircle and three excircles constructed.
45 Degree Triangle
Triangles are made of various substances such as wood, rubber, celluloid, and steel.
30-60 Degree Triangle
Triangles are made of various substances such as wood, rubber, celluloid, and steel.
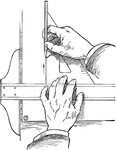
Triangle Scales
The triangle scale has six surfaces for different graduations, and the scales are arranged so that the…
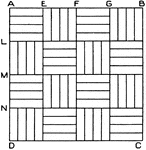
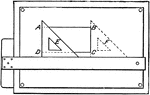
Square Exercise
Square pattern exercise: Divide A D and A B into 4 equal parts and draw horizontal and vertical lines.…
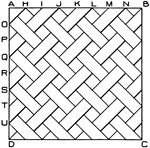
Weave Exercise
Weave pattern exercise: Divide A D and A B into 8 equal parts, and through the points O, P, Q, H, I,…

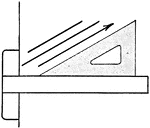
Drawing Parallel Lines Exercise
Parallel lines are drawn by using a triangle in combination with a T-square. To draw a line parallel…
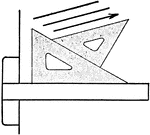
Drawing Perpendicular Lines Exercise
Lines perpendicular to each other may be drawn by using a triangle in combination with the T-square.…
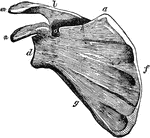
Scapula
Scapula. Labels: a, superior angle; d, the glenoid cavity, or socket for the round head of the arm bone;…
Tide Theory, Triangle
The figure shows a 30 degree triangle made from a square depicting the harmony and syzygy of tides in…

Tide Theory, Triangle
The figure shows a 30 degree triangle made from a square depicting the harmony and syzygy of tides in…
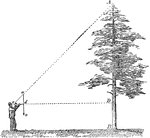
Measuring Tree Height
A man measuring the height of a tree by determining the angle and how far away he is standing.
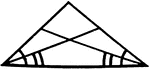
Angle Bisectors In An Isosceles Triangle
Illustration showing that if two angles of a triangle are equal, the bisectors of these angles are equal.
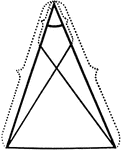
Equal Segments In An Isosceles Triangle
Illustration showing that if equal segments measured from the vertex are laid off on the arms of an…
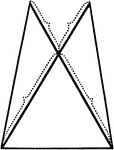
Equal Segments In An Isosceles Triangle
Illustration showing that if equal segments prolonged through the vertex are laid off on the arms of…
Base Angles In An Isosceles Triangle
Illustration showing that can be used to prove that the base angles of an isosceles triangle are equal.
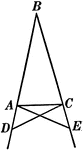
Equal Segments In An Isosceles Triangle
Illustration showing that if equal segments measured from the end of the base are laid off on the base…
Equal Segments In An Isosceles Triangle
Illustration showing that if equal segments measured from the end of the base prolonged are laid off…
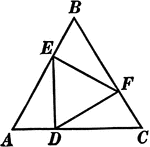
Equilateral Triangle Inscribed In An Equilateral Triangle
Illustration used to prove that triangle EFD is equilateral given that triangle ABC is equilateral and…
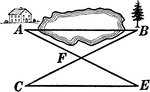
Using Triangles To Measure A Lake
Illustration that uses triangles to measure the distance across a lake.
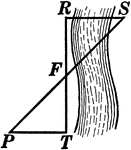
Using Triangles To Measure A River
Illustration that uses triangles to measure the distance across a river.
Using Triangles To Measure A River
Illustration that uses triangles to measure the distance across a river.
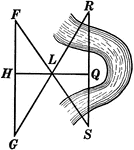
Using Triangles To Measure A River
Illustration that uses triangles to measure the distance across a river.
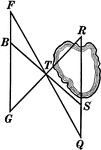
Using Triangles To Measure A Lake
Illustration that uses triangles to measure the distance across a lake.
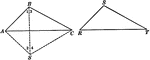
Equal Triangles
Illustration used to show that two triangles are equal if the three sides of one are equal respectively…
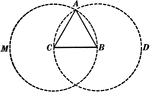
Construction Of Equilateral Triangle
Illustration used to show how to construct an equilateral triangle, with a given line as a side.
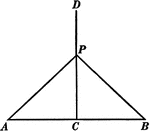
Triangle With Perpendicular Bisector
Illustration showing a perpendicular bisector of a triangle extended outside of the triangle.
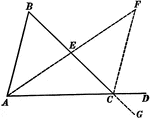
Exterior Angle of Triangle Theorem
Illustration used to prove that "If one side of a triangle is prolonged, the exterior angle formed is…
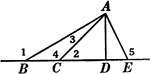
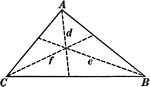
Segments and Angles in a Triangle
Illustration of a triangle with interior segments and angles labeled.
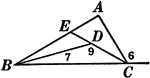
Segments and Angles in a Triangle
Illustration of a triangle with interior segments and angles labeled.
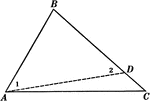
Sides of Triangle Theorem
Illustration used to prove that "If two sides of a triangle are unequal, the angle opposite the greater…
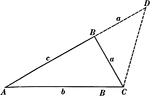
Sides of Triangle Theorem
Illustration used to prove that "The sum of any two sides of a triangle is greater than the third side."
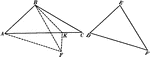
2 Triangles Theorem
Illustration used to prove that "If two triangles have two sides of one equal respectively to two sides…
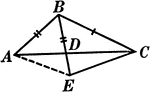
Segments Labeled In A Triangle
Illustration of triangle ABC with BE extended through the triangle at point D. Segment AB is equal to…
2 Triangles Theorem
Illustration used to prove that "If two triangles have two sides of one equal respectively to two sides…
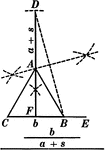
Construction Of An Isosceles Triangle
Illustration of the construction used to create an isosceles triangle, given the bases and the sum of…