Clipart tagged: ‘arcs’
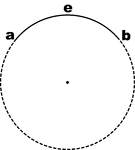
Golden Angle
Illustration showing the golden angle. The golden angle is the smaller of two angles created by dividing…
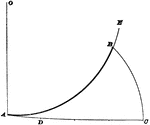
Construction Of Arc
Illustration used to show how to "find an arc of a circle having a known radius, which shall be equal…
Positive and Negative Arcs in Circles
Illustration showing arcs measured in positive and negative angles.
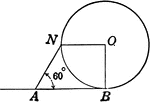
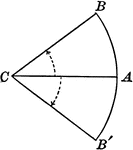
Construction Of Center
Illustration used to show how to find the center when given an arc and its radius.

Arc of Circle
An illustration of an arc of a circle. An arc is any part of the circumference of a circle.
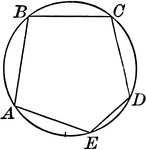
Circle with Arc, Chord, Inscribed Angle, Circumscribed Polygon
Illustrations of a circle with arc, chord, inscribed angle, and circumscribed about a polygon.
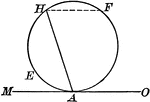
Circle With Chord and Tangent
Illustration of a circle showing an angle included by a tangent and a chord drawn from the point of…
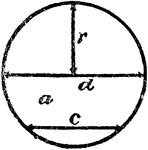
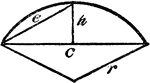
Radius, Diameter, and Chord In A Circle
An illustration showing a circle with radius r, diameter d, and chord c.
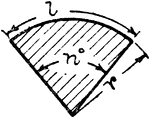
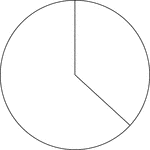
Sector Of Circle
Illustration of a sector of a circle. A sector is the space between an arc and two radii drawn to the…
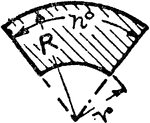
Sector Of A Hollow Circle
Illustration of a sector of a hollow circle. A sector is the space between an arc and two radii drawn…
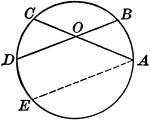
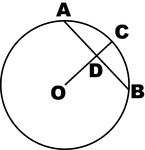
Circle With Two Intersecting Chords
Illustration of a circle with two intersecting chords within the circumference. The angle formed is…
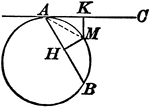
Circle With a Tangent Line and Chord
Illustration showing that from any point in the circumference of a circle, a chord and a tangent are…
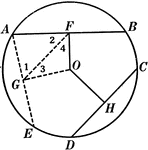
Unequal Chords in Equal Circles Theorem
Illustration used to show that "In equal circles, or in the same circle, if two chords are unequal,…
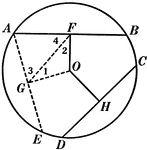
Unequal Chords in Equal Circles Theorem
Illustration used to show that "In equal circles, or in the same circle, if two chords are unequal,…
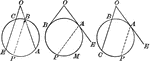
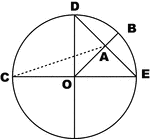
Circles With Angles Formed by Secants and Tangents
Illustration showing angles formed by two secants, two tangents, or a tangent and a secant, drawn to…
Intersecting Lines in Circles
Illustration showing various circles and the angles formed by intersecting lines.
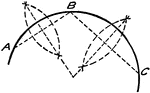
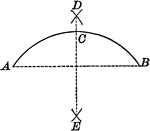
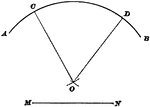
Draw Circular Arc Through Three Given Points
"Join AB and BC, bisect AB and BC by perpendiculars. Their intersection will be the center of the required…
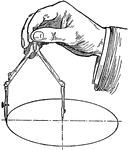
Inking a Circle
Always draw a circle in one stroke, inclining the compass in the direction of the line and rolling the…
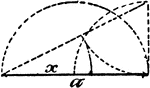
Model Of Geometric Proportions
An illustration showing a model that illustrates the following relationships: a:x = x:a - x, x = √(a²…
Byzantine Interlacement Band
The Byzantine interlacement band consists of wavy arcs and curves that have an angular bend. This design…
Romanesque Interlacement Band
The Romanesque interlacement band consists of wavy arcs and curves that have an angular bend. It is…
Romanesque Interlacement Band
The Romanesque interlacement band consists of wavy arcs and curves that have an angular bend.
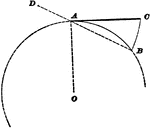
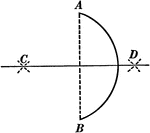
Construction Of Line Equal To Arc
Illustration used to show how to find a straight line of the same length as a given arc of a circle.

Hindley's Screw
"A screw cut on a solid, of such form that if any plane be taken through its longitudinal axis, the…
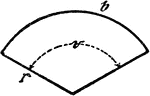
Circle Sector
An illustration showing a circle sector with radius r, center/central angle v, and length of circle…
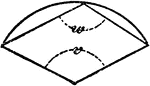
Circle Sector
An illustration showing a circle sector with center/central angle v and polygon angle w.
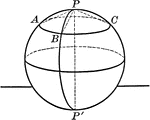
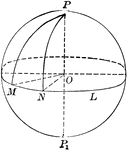
Sphere With Arcs and Poles
A sphere with arcs and poles. "The distance of all points in the circumference of a circle of a sphere…
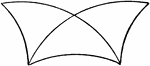
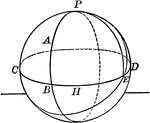
Superimposed Symmetrical Spherical Triangles
Diagram showing 2 superimposed symmetrical spherical triangles.