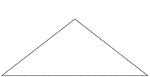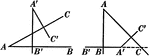
Angles With Perpendicular Sides Are Equal or Supplementary Proof
Illustration used to prove "Two angles whose sides are perpendicular each to each are either equal or…

Exterior Angle Proof
Illustration used to prove "An exterior angle of a triangle is equal to the sum of the two remote interior…
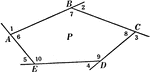
Sum of Exterior Angles of a Polygon Proof
Illustration used to prove "If the sides of any polygon are prolonged in succession one way, no two…
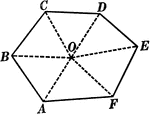
Sum of Interior Angles of a Polygon Proof
Illustration used to prove "The sum of all the angles of any polygon is twice as many right angles as…
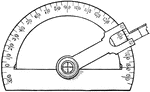
Semicircular Protractor
A 6 inches diameter protractor to measure angles to half degrees, made of brass or German silver. They…
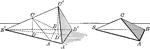
Triangular Pyramids for Volume
Illustration showing that the volume of 2 triangular pyramids, having the same trihedral angle of the…
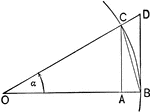
Triangles and Sectors in Quadrant I
Illustration of an angle &alpha with the vertex at the center, O, of a circle with radius OB. AC and…
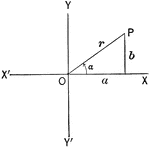
Triangle in Quadrant I
Illustration of an angle &alpha with the terminal side used to draw a triangle in quadrant I.
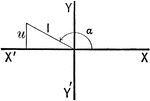
Triangle in Quadrant II
Illustration of an angle with the terminal side used to draw a triangle in quadrant II.
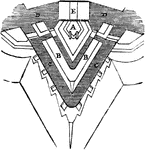
Ravelin
A detached triangular work in fortification, with two embankments which form a projecting angle. In…

Redans
In field fortification, the simplest kind of work employed, consisting of two parapets of earth raised…

Law of Reflected Motion
"The angle, ABD, included between the direction of the moving body before it strikes the reflecting…
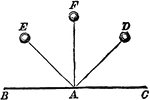
Reflected Motion
"... if a ball be thrown from F against the surface B C so as to strike it perpendicularly at A, it…
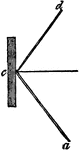
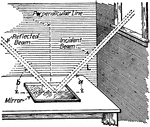
Reflection of Light
"The ray a, c, is the ray of incidence, and that from c, to d, is the ray or reflection. The angles…
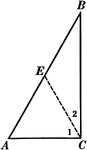
Relationships In A Right Triangle
Illustration used to show "If one acute angle of a right triangle is double the other, the hypotenuse…
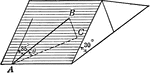
Roof With 30 degree Inclination for Trigonometry Triangle Problems
Roof with a slope of 30 degrees. Angle theta is the inclination to the horizontal of the line AB, drawn…
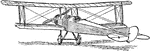
Airplane Rudder and Elevator
A propeller airplane with rudder, elevator, and ailerons. The rudder stabilizes the plane vertical,…
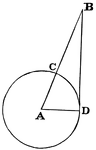
Circle with Secant
A secant is "a line which cuts a figure in any way. Specifically, in trigonometry, a line from the center…
Shoe Block
"Nautically, a block with two sheaves, whose axes are at right angles to each other, used for the buntlines…
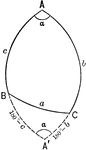
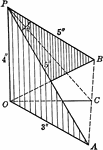
Law of Sines
Illustration two types of triangles that can be used to model the law of sines. "In a plane triangle…
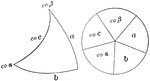
Napier's Right Spherical Triangle
Illustration of a right spherical triangle and the five circular parts placed in the sectors of a circle…
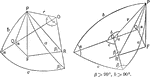
Relationships In A Spherical Triangle
Illustration used, with the law of sines, to find the relation between two sides of a spherical triangle…
Relationships In A Spherical Triangle
Illustration used, with the law of cosines, to find the relation between the three sides and an angle…
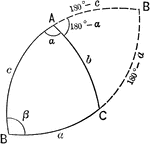
Relationships In A Spherical Triangle
Illustration used to extend the law of cosines when finding the relation between the three sides and…
Relationships In A Spherical Triangle
Illustration used to extend the law of cosines when finding the relation between the three sides and…
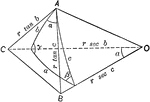
Right Spherical Triangle
Illustration of a right spherical triangle with a and b the sides, and α and β the angles…
Two Squares With Sides of Lengths 3,4,5 Placed at Right Angles to Each Other
Two set squares, whose sides are 3,4, and 5 in., are placed so that their 4-in. sides and right angles…
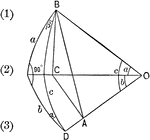
Survey of Lake Front in Chicago
Illustration showing a survey on the lake front in Chicago with distances and angle measures shown.

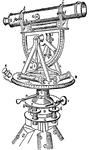
Theodolite
"An instrument for the measurement of horizontal angles, consisting essentially of a telescope mounted…
Theodolite
"An instrument much employed in land-surveying for the measurement of angles horizontal and verticle."…
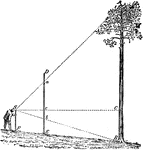
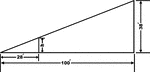
Using Proportions To Find Heights of Trees
Triangle diagram for measuring heights of trees using proportions.

Using Proportions To Find Heights of Trees
Triangle diagram for measuring heights of trees using proportions
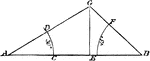
Construction of Triangle Given Angles and Base
Illustration used to construct a triangle given the length of the base and the two base angles.
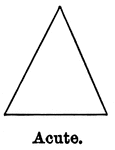
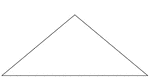
Acute Triangle
Illustration showing an acute triangle (one that has all three angles that are acute).
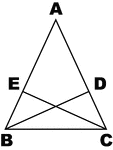
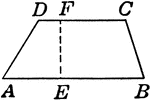
Altitudes Drawn from Base Angles of Isosceles Triangles
Illustration to show altitudes drawn from base angles of an isosceles triangle.
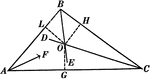
Angle Bisectors In A Triangle
Illustration used to prove "The bisectors of the angles of a triangle are concurrent in a point which…
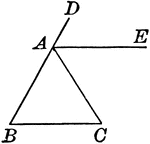
Bisector of an Exterior Angle of an Isosceles Triangle
Illustration to show that the bisector of an exterior angle of an isosceles triangle, formed by producing…
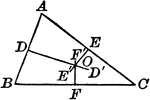
Circumcenter of Triangle
Illustration to show perpendicular bisectors in a triangle. It is known as the circumcenter.

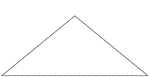
Equiangular Triangle
Illustration showing an equiangular triangle (one that has all three angles that are equal).
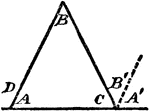
Exterior And Interior Angles Of A Triangle
An illustration showing a triangle with interior angles A, B, C, and exterior angles D, and A' + B'.
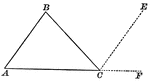
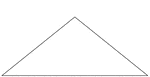
Triangle with Exterior Angles Drawn
Illustration showing a triangle with exterior angles. This can be used to show the sum of the three…
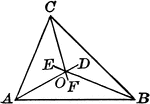
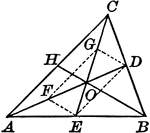
Incenter of Triangle
Illustration to show angle bisectors in a triangle. It is known as the incenter.