Shaft lining
"Until hard rock is reached the sides of the excavation are supported temporarily by a lining"—Finley,…
Shapes
Four different types of forms or shapes: right triangle, isosceles triangle, rectangle, and circle.

Flanch Shield
"The Flanch is formed of a segment of a circle placed on the side of the shield. It is always borne…

Rustre Shield
"The Rustre differs from the mascle in that the perforation of the device is circular and not throughout."—Aveling,…
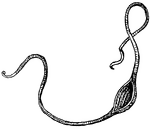
Sling
"An instrument for throwing stones or bullets, consisting of a strap and two strings attached to it.…
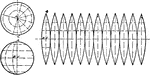
Development of Sphere Gore Method
An illustration in flattening the sphere using Gore method by creating cylinder sections with equal…
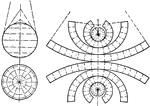
Development of Sphere Zone Method
An illustration of a development of sphere using Zone method by creating sections of rolled out cones.
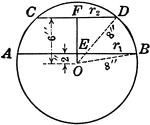
Sphere With 8-inch Diameter Cut by Planes
An 8-inch sphere cut by parallel planes, one 2 inches from center and the other 6 inches from center.…
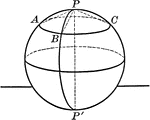
Sphere With Arcs and Poles
A sphere with arcs and poles. "The distance of all points in the circumference of a circle of a sphere…
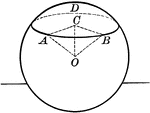
Circle Made by Plane Passing Through a Sphere
"Every section of a sphere made by a plane is a circle."
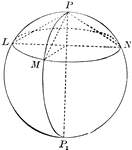
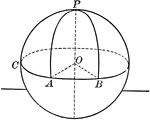
Circumference of a Circle of a Sphere
Diagram used to prove the theorem: "All points in the circumference of a circle of a sphere are equally…
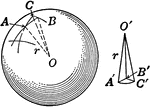
Sphere Cut Into Pyramids.
An illustration of a sphere cut into polygons as bases with their vertices at the center of sphere.
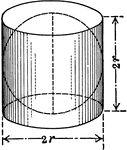
Sphere Inside of Cylinder.
An illustration of a sphere inside of a cylinder with radius/diameter labeled. Illustration for showing…
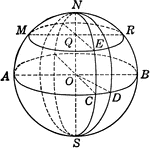
Sphere with arcs and circles labeled.
An illustration of a sphere showing diameter, arcs, and circles. A sphere is a solid bounded by a curved…
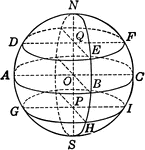
Sphere With Arcs, Circles, and Zones Labeled.
An illustration of a sphere showing diameter, arcs, and circles. A sphere is a solid bounded by a curved…

Sphere Made by Revolution of Semicircle
Illustration of a sphere generated by the revolution of a semicircle ACB about its diameter AB as an…

Sphere With Tangent Plane
Illustration of a sphere with a tangent plane. "A plane perpendicular to a radius at its extremity is…
Sphere With Tangent Plane
Illustration of a sphere with a tangent plane. "A plane perpendicular to a radius at its extremity is…
Polyhedral Angle in a Sphere
Illustration of a sphere with a polyhedral angle whose vertex is at the center of the sphere and whose…
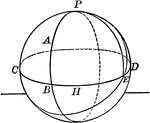
Sphere With Quadrants and Poles
A sphere with quadrants and poles. "A point on the surface of a sphere, which is at the distance of…
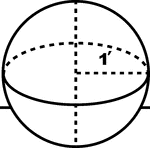
Sphere With a Radius of 1 foot
An illustration of a sphere showing a radius of 1 foot. Illustration could be used for calculating volume.
Wooden Sphere
A wooden hollow sphere created by using a lathe and wooden templet at a desired diameter.
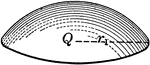
Zones or Segments of Spheres
An illustration of a zone of a sphere. A zone occurs when a sphere is cut by parallel planes that are…
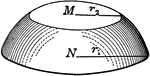
Zones or Segments of Spheres
An illustration of a zone of a sphere. A zone occurs when a sphere is cut by parallel planes that are…

Equal Spheres With Triangles
Illustration of equal spheres with equilateral and equiangular triangles.
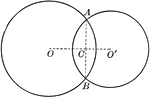
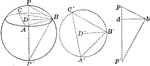
Intersecting Spheres
Illustration of two intersecting spheres. "The intersection of two spherical surfaces is the circumference…
Flanged Spherical Segment
An illustration of a flanged spherical segment with a diameter of 10 inches. Illustration could be used…
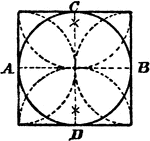
Construction Of A Square Circumscribed About A Circle
An illustration showing how to construct a square circumscribed about a circle. "Draw the diameters…
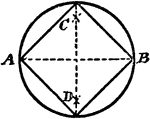
Construction Of A Square Inscribed In A Circle
An illustration showing how to construct a square inscribed in a circle. "Draw the diameter AB, and…
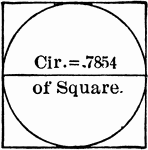
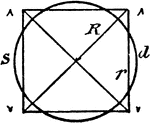
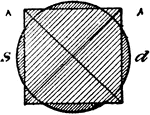
Circle Inscribed In A Square
An illustration of a circle inscribed in a square. It can be used to show that the area of a circle…
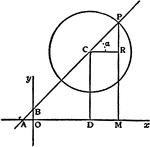
Straight Line
The straight line is the simplest type of locus and the simplest first degree equation.
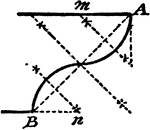
Construction Of A Talon
An illustration showing how to construct a talon, or two circle arcs that will tangent themselves, and…
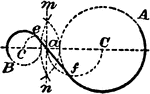
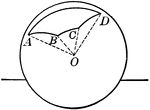
Construction Of Tangent Between 2 Circles
An illustration showing how to construct a tangent between 2 given circles. "Join the centers C and…
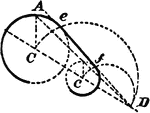
Construction Of Tangent To 2 Circles
An illustration showing how to construct a tangent to 2 given circles of different diameters. "Join…
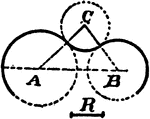
Construction Of Circle Tangent To 2 Circles
An illustration showing how to construct a tangent circle to 2 given circles. "Join centers C and c…
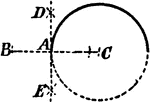
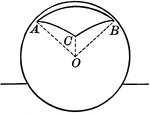
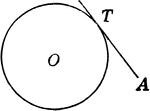
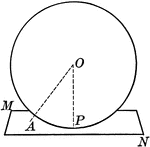
Construction Of Tangent To Circle
An illustration showing how to construct a tangent to a circle through a given point in a circumference.…
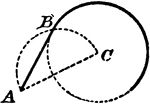
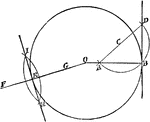
Construction Of Tangent To Circle
An illustration showing how to construct a tangent to a circle through a given point outside of a circumference.…
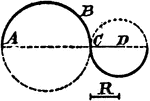
Construction Of Circle Tangent To Circle
An illustration showing how to construct a tangent circle to a circle with a given radius. "Through…
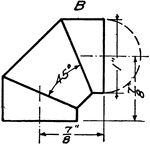
Development Exercise of Cylinder using Three Piece Elbow
The exercise problem of creating a three piece elbow development or rolled out image of the cylinder…
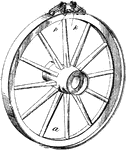
Adjustable Tire
A tire is ring shaped, the earliest tires were bands of iron placed on wooden wheels which were used…
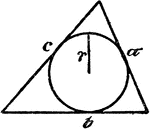
Circle Inscribed In A Triangle
An illustration showing a circle with radius r inscribed in a triangle with sides a, b, and c.
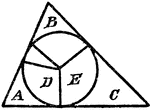
Circle Inscribed In A Triangle
An illustration showing a circle with sectors D and E inscribed in a triangle with angles, A, B, and…
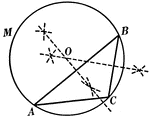
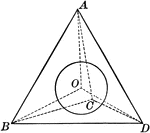
Construction Used to Circumscribe a Circle About a Triangle
Illustration used to circumscribe a circle about a given triangle.
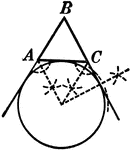
Construction Used to Escribe a Circle to a Triangle
Illustration used to escribe a circle to a given triangle. "A circle which is tangent to one side of…