Ancient Mariner
From Coleridge's The Rime of the Ancient Mariner. An old mariner tells his tales to a young man who…
Reindeer age articles, Bone points and needles
Bone points and bone needles. Crafted during the Reindeer age.
!["The part of the body in which the centre of gravity is situated, may be found, in some cases, by balancing it on a point. Thus the centre of gravity of the poker represented [here] lies directly over the point on which it is balanced." —Quackenbos 1859](https://etc.usf.edu/clipart/36300/36326/centergrav_36326_mth.gif)
Center of Gravity
"The part of the body in which the centre of gravity is situated, may be found, in some cases, by balancing…
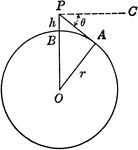
Circle With Center o and Radius r with point P
Circle modeling the earth. O is the center of the earth, r the radius of the earth, and h the height…
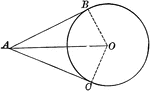
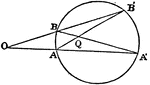
Circle With Two Tangents Drawn From an External Point
Illustration of a circle which illustrates that the tangents to a circle drawn from an external point…
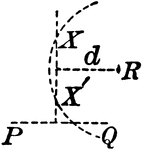
Construction of a Circle When Given Two Points and a Radius
Illustration used to construct a circle when given two points that it passes through and a radius.
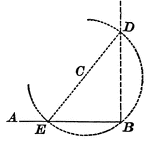
Construction of Perpendicular From a Given Point on a Straight Line
Illustration of the construction of a perpendicular to a line when given a point O on the straight line.
Construction of Perpendicular From a Given Point on a Straight Line
Illustration of the construction of a perpendicular to a line when given a point B on the straight line.
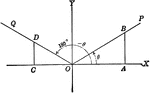
Coordinate Axis With Angles, Lines, and Perpendiculars Drawn
Coordinate axis with angle XOP equal to theta, Θ, and angle XOQ=180 - Θ. From any point in the terminal…
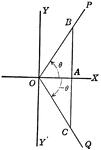
Coordinate Axis With Perpendiculars Drawn To Form Similar Right Triangles From Positive and Negative Theta, Θ
Angle XOP=Θ and angle XOQ=- Θ. From a point in the terminal side of each a perpendicular line is drawn…
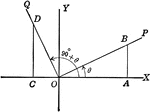
Coordinate Axis With Perpendiculars Drawn To Form Similar Right Triangles
Angle XOP=Θ and angle XOQ=90+Θ. From a point in the terminal side of each a perpendicular line is…
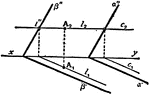
Curve in Pavement of Road
Illustration of blueprint used by highway engineers to widen the pavement on the inside of the curve…

Double Arrow, Black and White
The Double Arrow sign may be used to advise road users that traffic is permitted to pass on either side…

Double Arrow, Color
The Double Arrow sign may be used to advise road users that traffic is permitted to pass on either side…
Double Arrow, Outline
The Double Arrow sign may be used to advise road users that traffic is permitted to pass on either side…

Double Arrow, Silhouette
The Double Arrow sign may be used to advise road users that traffic is permitted to pass on either side…
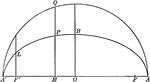
Ordinate and Major Axis of Ellipse
Illustration of half of an ellipse. The square of the ordinate of a point in an ellipse is to the product…
Auxiliary Circle and Hyperbola
Illustration of a hyperbola and its auxiliary circle. "Any ordinate of a hyperbola is to the tangent…
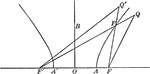
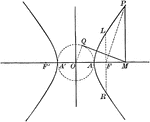
Foci Distance of Hyperbola
Illustration of a hyperbola with distances to foci drawn. "The difference of the distances of any point…
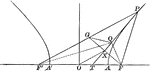
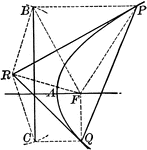
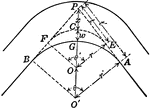
Line Bisecting Angle Between Focal Radii in Hyperbola
Illustration of a hyperbola with a line bisecting the focal radii. "If through a point P of an hyperbola…
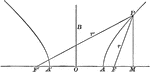
Point on a Hyperbola
Illustration of a point on a hyperbola. "If d denotes the abscissa (x-coordinate) of a point of an hyperbola,…
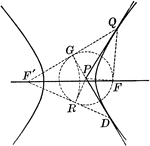
Tangent to Hyperbola
Illustration showing how to draw a tangent to an hyperbola from a given point P on the convex side of…
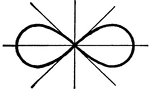
Lemniscate
A Lemniscate is, in general, a curve generated by a point moving so that the product of its distances…

Lincoln Cathedral
"Sectional compartment of the Nave of Lincoln Cathedral." — Encyclopedia Britanica, 1893

Lincoln Cathedral
"Sectional compartment of the Choir of Lincoln Cathedral." — Encyclopedia Britanica, 1893
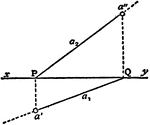
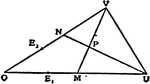
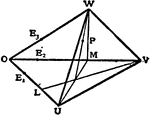
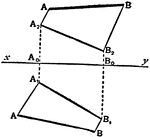
Line Projection
To find the projections of a line which joins two points, A, B given by their projections.
Straight Line With Points Divided Into Equal Parts
An illustration of a straight line with 5 points dividing it into 4 equal parts. Multiple of a given…
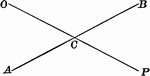
Intersecting Straight Lines
Illustration of two lines intersecting at a point. This can be used to show vertical angles.
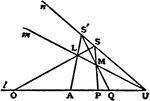
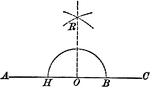
Lines Drawn From the Same Point in a Perpendicular to a Given line, Cutting Off Segments
Illustration showing two straight lines drawn from the same point in a perpendicular to a given line,…
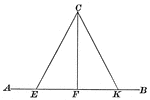
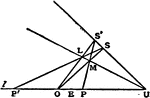
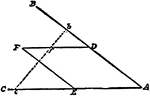
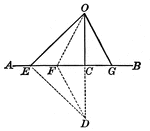
Lines Drawn to Another Line to Form Triangle
Illustration of two straight lines drawn from a point in a perpendicular to a given line, cutting off…

Man Sitting & Child Standing Near Lake
An illustration of a man sitting on the shore of a lake and a child standing behind him pointing into…
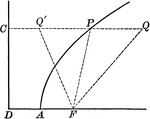
Point on Parabola
Illustration of a parabola showing point P is equidistant to the focus and the directrix.
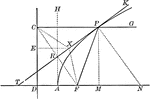
Point on Parabola
Illustration of a parabola. "If a line PT is drawn from any point P of the curve, bisecting the angle…
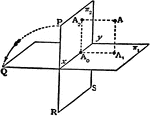
Perpendicular Planes
Two planes lie perpendicular to one another. A line perpendicular to plane 1 and a line perpendicular…
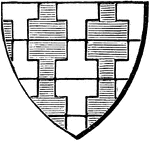
Potent Counter-Potent Shield
"Potent counter-potent, sometimes called varry cuppy, differs from potent in that the potents of the…
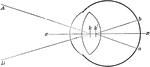
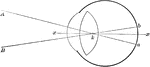
Retina
"Diagram illustrating the points at which incident rays meet the retina. xx, optic axis; k, first nodal…

Skadi Chooses a Husband
Skadi is a giantess and daughter of Thiassi who was killed while chasing Loki because of a trick. Skadi…