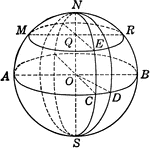
Sphere with arcs and circles labeled.
An illustration of a sphere showing diameter, arcs, and circles. A sphere is a solid bounded by a curved…
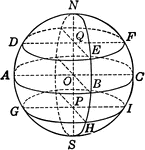
Sphere With Arcs, Circles, and Zones Labeled.
An illustration of a sphere showing diameter, arcs, and circles. A sphere is a solid bounded by a curved…
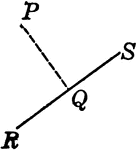
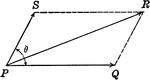
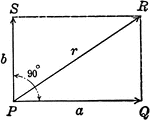
Perpendicular Line Drawn From Point P to Line RS
A perpendicular line drawn from point P to straight line RS.
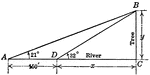
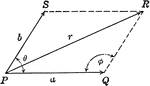
Right Triangle For Finding Distance Across a River
Right triangle ABC with angles A, B, C to be used for finding distance across a river. This is a trigonometry…
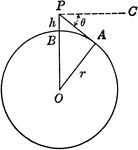
Circle With Center o and Radius r with point P
Circle modeling the earth. O is the center of the earth, r the radius of the earth, and h the height…
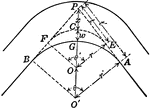
Curve in Pavement of Road
Illustration of blueprint used by highway engineers to widen the pavement on the inside of the curve…
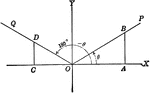
Coordinate Axis With Angles, Lines, and Perpendiculars Drawn
Coordinate axis with angle XOP equal to theta, Θ, and angle XOQ=180 - Θ. From any point in the terminal…
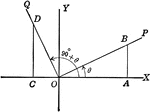
Coordinate Axis With Perpendiculars Drawn To Form Similar Right Triangles
Angle XOP=Θ and angle XOQ=90+Θ. From a point in the terminal side of each a perpendicular line is…
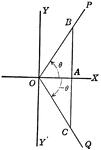
Coordinate Axis With Perpendiculars Drawn To Form Similar Right Triangles From Positive and Negative Theta, Θ
Angle XOP=Θ and angle XOQ=- Θ. From a point in the terminal side of each a perpendicular line is drawn…
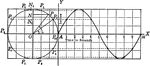
Projection of Points in Circular Motion
Illustration of the projection of point P as it moves around a vertical circle of radius 3 in. in a…
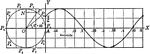
Projection of Points in Circular Motion
Illustration of the projection of point P as it moves around a vertical circle of radius 2 ft. in a…
Resultant Vectors
Illustration of the resultant vector when two vectors are acting upon a body at point P.
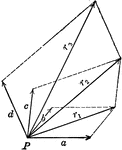
Resultant Vector From 4 Forces
Illustration of the resultant vector when four vectors are acting upon a body at point P.
Resultant Vector With Vectors at 90 degrees
Illustration of the resultant vector when two vectors are acting upon a body at point P at 90 degrees.
Resultant Vector With Vectors at Angle Ǝ
Illustration of the resultant vector when two vectors are acting upon a body at point P at Ǝ degrees.
Detection of Astigmatism
Lines for the detection of astigmatism. "The refracting surfaces of the eye acting together are equivalent…
Larynx
The larynx viewed from its pharyngeal opening. The back wall of the pharynx has been divided and its…
Straight Line With Points Divided Into Equal Parts
An illustration of a straight line with 5 points dividing it into 4 equal parts. Multiple of a given…
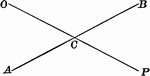
Intersecting Straight Lines
Illustration of two lines intersecting at a point. This can be used to show vertical angles.
Lines Drawn to Another Line to Form Triangle
Illustration of two straight lines drawn from a point in a perpendicular to a given line, cutting off…
Perpendicular Line Drawn to a Given Line from an External Point
Illustration showing only one perpendicular can be drawn to a given line from a given external point.
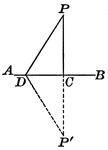
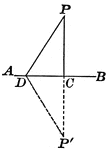
Perpendicular Line Drawn To a Given Line From an External Point
Illustration showing that the perpendicular is the shortest line that can be drawn to a straight line…
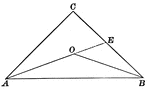
Lines Drawn From the Point C to the Extremities of the Straight line AB
Illustration showing two lines CA and CB drawn from the point C to the extremities of the straight line…
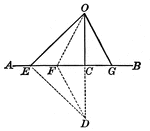
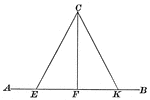
Lines Drawn From the Same Point in a Perpendicular to a Given line, Cutting Off Segments
Illustration showing two straight lines drawn from the same point in a perpendicular to a given line,…
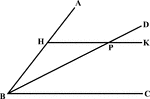
Angle Bisector Drawn Parallel to A Side
Illustration to show that if through any point in the bisector of an angle a line is drawn parallel…