Clipart tagged: ‘Right Triangle’

Angles Used to Illustrate Sum and Difference of Two Angles
Angles used to illustrate the sum and difference of two angles and trig identities.
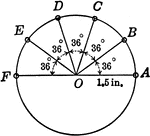
Circle With 36 degree Angles and Radius 1.5 in.
Circle with 36 degree angles marked. This diagram can be used with the following trig problem: Locate…
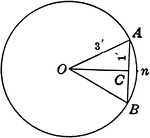
Circle With a Chord of 2 ft. and a Radius of 3 ft.
Circle with chord AB=2 ft. and radius OA = 3 ft.. Triangle AOC is a right triangle. Angle AOC=half angle…
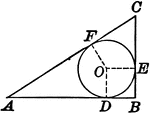
Circle Inscribed in a Right Triangle
Illustration showing the diameter of a circle inscribed in a right triangle is equal to the difference…
Circle With Inscribed Right Angle in Semicircle
Illustration of a circle with a right angle inscribed in a semicircle.
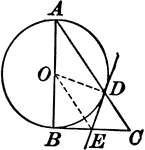
Circle With a Right Triangle
Illustration where one leg of a right triangle is the diameter of a circle. The tangent at the point…
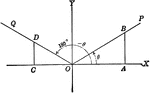
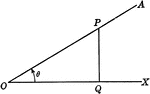
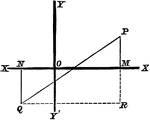
Coordinate Axis With Angles, Lines, and Perpendiculars Drawn
Coordinate axis with angle XOP equal to theta, Θ, and angle XOQ=180 - Θ. From any point in the terminal…
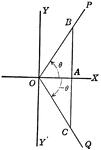
Coordinate Axis With Perpendiculars Drawn To Form Similar Right Triangles From Positive and Negative Theta, Θ
Angle XOP=Θ and angle XOQ=- Θ. From a point in the terminal side of each a perpendicular line is drawn…
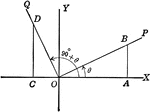
Coordinate Axis With Perpendiculars Drawn To Form Similar Right Triangles
Angle XOP=Θ and angle XOQ=90+Θ. From a point in the terminal side of each a perpendicular line is…
Forms
Showing different types of forms or shapes: rectangle, right triangle, acute triangle, and obtuse triangle.
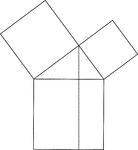
Euclid's Pythagorean Theorem Proof
Illustration used to prove the Pythagorean Theorem, according to Euclid. A perpendicular is drawn from…
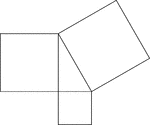
Geometric Pythagorean Theorem Proof
Illustration that can be used to prove the Pythagorean Theorem, the sum of the squares of the legs is…
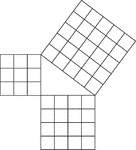
Geometric Pythagorean Theorem Proof
Illustration that can be used to prove the Pythagorean Theorem, the sum of the squares of the legs is…
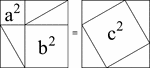
Pythagorean Theorem Proof by Rearrangement
A visual illustration used to prove the Pythagorean Theorem by rearrangement. When the 4 identical triangles…
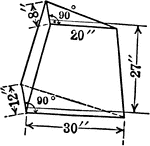
Pyramid Frustum With Triangular Bases and Height of 27 inches
An illustration of a pyramid with the top cut off by a plane parallel to the base. The remaining part…
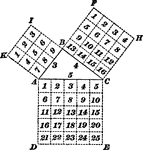
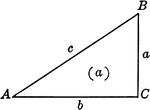
Right Triangle
"In any right triangle, the square described on the hypotenuse is equal to the sum of the squares described…

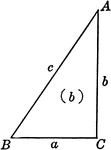
Right Triangle
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…

Right Triangle
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…
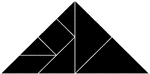
Right Triangle
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…
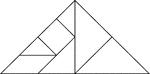
Right Triangle
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…
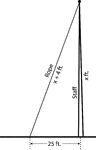
Right Triangle Formed by Flagpole (x feet high) and Ground With Rope (x+4 feet)
An illustration of a right triangle made up of a flagpole, rope, and the ground. Illustration could…
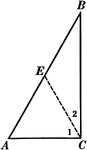
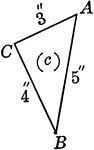
Relationships In A Right Triangle
Illustration used to show "If one acute angle of a right triangle is double the other, the hypotenuse…
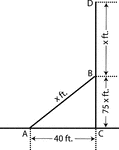
Right Triangle With Sides x, 75, and 40
An illustration of a right triangle with sides, 40, 75, and x. Additional height of x is added to 75.…
Roof With 30 degree Inclination for Trigonometry Triangle Problems
Roof with a slope of 30 degrees. Angle theta is the inclination to the horizontal of the line AB, drawn…
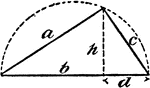
Right Triangle Inscribed In A Semicircle
An illustration of a right triangle inscribed in a semicircle.
Shapes
Four different types of forms or shapes: right triangle, isosceles triangle, rectangle, and circle.
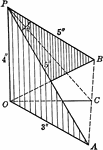
Two Squares With Sides of Lengths 3,4,5 Placed at Right Angles to Each Other
Two set squares, whose sides are 3,4, and 5 in., are placed so that their 4-in. sides and right angles…
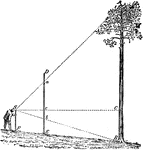
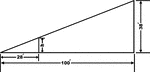
Using Proportions To Find Heights of Trees
Triangle diagram for measuring heights of trees using proportions.

Using Proportions To Find Heights of Trees
Triangle diagram for measuring heights of trees using proportions
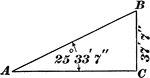
Triangle With Angle of 25 ° 33' 7" and Side of 37' 7"
Right triangle with angle of 25 ° 33' 7" and side of 37' 7"
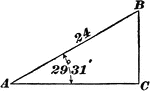
Triangle With Angle of 29 ° 31' and Side of 24
Right triangle with angle of 29 ° 31' and side of 24.
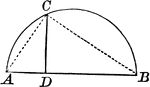
Right Triangle Inscribed in Semicircle Shows Mean Proportional
Right triangle inscribed in semicircle. Illustration shows that the perpendicular from any point in…
Right Triangle
Illustration of a right triangle used to show the Pythagorean Theorem (the square of the hypotenuse…
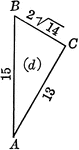
Right Triangle with leg 13 and hypotenuse 15
Right triangle ABC with angles A, B, C labeled and leg of 13 with hypotenuse of 15.
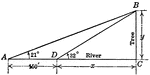
Right Triangle For Finding Distance Across a River
Right triangle ABC with angles A, B, C to be used for finding distance across a river. This is a trigonometry…
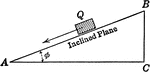
Inclined Plane Forming Right Triangle
Inclined plane forming right triangle showing the velocity of a body sliding a distance,s, down a smooth…
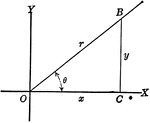
Right Triangle OCB With, x, y, and r shown
Right triangle OCB that can be used to show the relationships between x, y, r, and Θ.
Right Triangle Showing Pythagorean Theorem
Illustration of a right triangle that can be used to show Pythagorean theorem. "In any right triangle,…
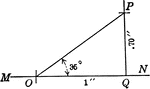
Right Triangle With Sides .7 and 1 and Angle of 35 degrees
Right triangle OQP with angle of 35 degrees, height of .70 inches, and leg of 1 inch.
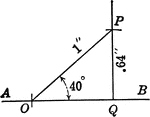
Right Triangle With Sides .64 and 1 and Angle of 40 degrees
Right triangle OQP with angle of 40 degrees, height of .64 inches, and hypotenuse of 1 inch.
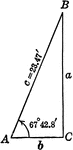
Right Triangle ABC With Angle 67 degrees 42.8 minutes and Hypotenuse 23.47 ft.
Right triangle ABC with a base angle of 67 degrees 4208 minutes and a hypotenuse of 23.47 ft.
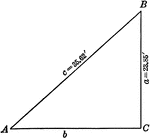
Right Triangle ABC With Leg 23.85 ft. and Hypotenuse 35.62 ft.
Right triangle ABC with a leg of 23.85 feet and a hypotenuse of 35.62 feet.
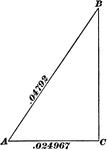
Right Triangle With Side .024967 and Hypotenuse .04792
Right triangle with side .024967 and hypotenuse .04792.
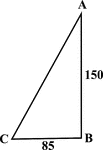
Right Triangle With Sides 85 and 150
Illustration of a right triangle with legs labeled 150 and 85. This could be used to find the hypotenuse…