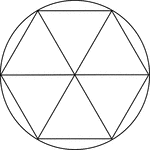
Regular Hexagon Inscribed In A Circle
Illustration of a regular hexagon inscribed in a circle. This can also be described as a circle circumscribed…
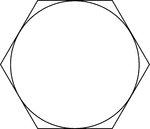
Regular Hexagon Circumscribed About A Circle
Illustration of a regular hexagon circumscribed about a circle. This can also be described as a circle…
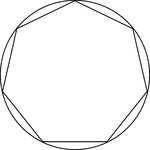
Regular Heptagon/Septagon Inscribed In A Circle
Illustration of a regular heptagon/septagon inscribed in a circle. This can also be described as a circle…
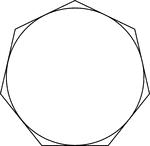
Regular Heptagon/Septagon Circumscribed about a Circle
Illustration of a regular heptagon/septagon circumscribed about a circle. This can also be described…
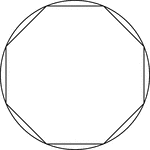
Regular Octagon Inscribed In A Circle
Illustration of a regular octagon inscribed in a circle. This can also be described as a circle circumscribed…
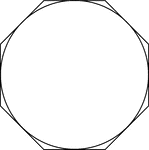
Regular Octagon Circumscribed About A Circle
Illustration of a regular octagon circumscribed about a circle. This can also be described as a circle…
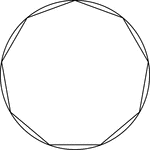
Regular Nonagon Inscribed In A Circle
Illustration of a regular nonagon inscribed in a circle. This can also be described as a circle circumscribed…
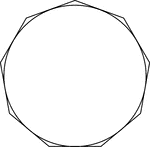
Regular Nonagon Circumscribed About A Circle
Illustration of a regular nonagon circumscribed about a circle. This can also be described as a circle…
Cyclic Pentagon
Illustration of a cyclic pentagon, a pentagon inscribed in a circle. This can also be described as a…
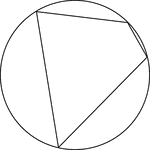
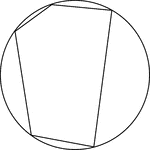
Cyclic Quadrilateral
Illustration of a cyclic quadrilateral, a quadrilateral inscribed in a circle. This can also be described…
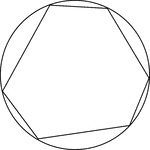
Cyclic Hexagon
Illustration of a cyclic hexagon, a hexagon inscribed in a circle. This can also be described as a circle…
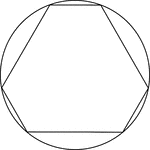
Cyclic Hexagon
Illustration of a cyclic hexagon, a hexagon inscribed in a circle. This can also be described as a circle…
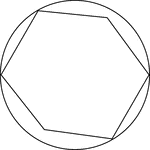
Hexagon In A Circle
Illustration of a hexagon in a circle. Four of the six vertices of the hexagon are bound by the circle…

Star Inscribed In A Circle
Illustration of a 5-point star inscribed in a circle. This can also be described as a circle circumscribed…
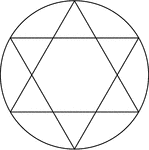
Star Inscribed In A Circle
Illustration of a 6-point star created by two equilateral triangles (often described as the Star of…
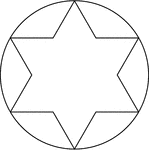
Star Inscribed In A Circle
Illustration of a 6-point star (convex dodecagon) inscribed in a circle. This can also be described…
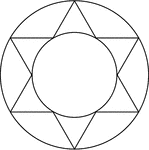
Star Inscribed And Circumscribed About Circles
Illustration of a 6-point star (convex dodecagon) inscribed in a large circle and circumscribed about…
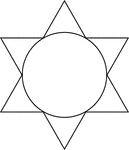
Star Circumscribed About A Circle
Illustration of a 6-point star (convex dodecagon) circumscribed about a circle. This can also be described…
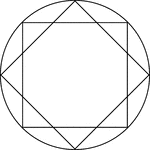
Star Inscribed In A Circle
Illustration of an 8-point star, created by two squares at 45° rotations, inscribed in a circle.…
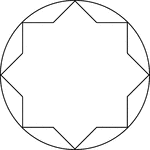
Star Inscribed In A Circle
Illustration of an 8-point star, or convex polygon, inscribed in a circle. This can also be described…
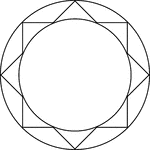
Star Inscribed And Circumscribed About Circles
Illustration of an 8-point star (convex polygon) inscribed in a large circle and circumscribed about…
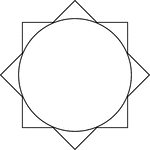
Star Circumscribed About A Circle
Illustration of an 8-point star (convex polygon) circumscribed about a circle. This can also be described…

Triangle With Circle Constructions
Illustration of a triangle with its incircle and three excircles constructed.

Beam Compasses
For larger circles beam compasses are used. The two parts called channels which carry the pen and the…
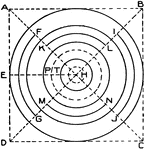
Circle Exercise
Circle pattern exercise: Draw diagonals A C and D B, and with the T-square draw the line E H. Now mark…
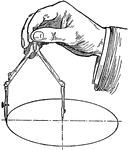
Inking a Circle
Always draw a circle in one stroke, inclining the compass in the direction of the line and rolling the…

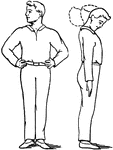
Exercise
"Hands on hips. Twist the trunk around in a circle, first one way, then the other." -Foster, 1921
Exercise
"Tense the muscles of the neck and turn head... forward and back and around in a circle." -Foster, 1921
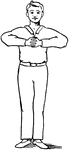
Exercise
"Arms outstretched horizontally. Make the hands describe a full circle forward and back." -Foster, 1921
Exercise
"Arms outstretched horizontally. Make the hands describe a full circle forward and back." -Foster, 1921

Girl
A little girl sitting among flowers. The circle is bordered with hand games and images of playing.
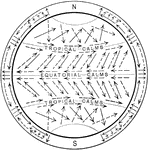
Atmospheric Circulation
"The inner circle incloses a map upon which the horizontal direction of the lower currents is shown…
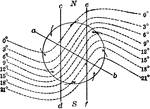
Isotherms in a Cyclone
The diagram "shows the isotherms in a cyclone of, say, 500 miles in diameter, as indicated by the circle,…
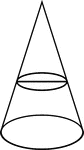
Conic Section Showing A Circle
Illustration showing the definition of a circle as a conic section. The section of a right circular…
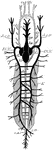
Teleostean Circulation
"Diagram of Teleostean circulation. A., auricle; V., ventricle; v.a., ventral aorta; a.br., afferent…
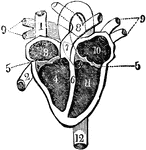
Heart and its Chambers
View of the heart with its several chambers exposed and the vessels in connection with them. Labels:…
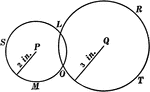
2 Intersecting Circles
Illustration showing a circle with a radius of 2 in. intersecting a circle with a radius of 3 in..
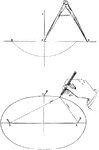
Construction Of An Ellipse
Illustrations used to construct an ellipse. Fig. 59 shows a compass at C used to strike a circle. Where…
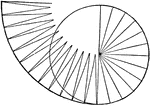
Circle Made Up Of Triangles
"A circle may be considered as made up of triangles whose bases form the circumference, and whose altitude…
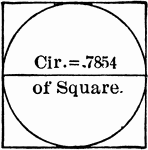
Circle Inscribed In A Square
An illustration of a circle inscribed in a square. It can be used to show that the area of a circle…
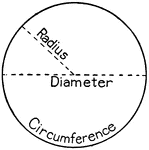
Circle Parts
A circle with labels for radius, diameter, and circumference. The visual will help to remember what…
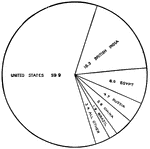
Pie Graph
A pie graph of "Proportion of World's Supply of Cotton contributed by each country" labeled with country…
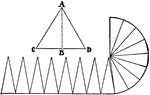
Area of Circle with Triangles
"In the triangle above, the line AB is its altitude. Since we know how to find the area of one triangle,…

Arc of Circle
An illustration of an arc of a circle. An arc is any part of the circumference of a circle.
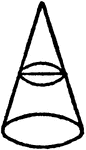
Conic Section Showing A Circle
An illustration showing the intersection of a plane and a cone. The cone is intersected by a plane parallel…
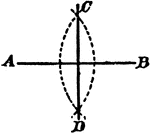
Construction Of A Line Divided In Equal Parts
An illustration showing the construction used to divide a line AB into two equal parts; and to erect…
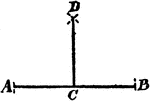
Construction Of A Perpendicular
An illustration showing the construction used to erect a perpendicular. "With C as a center, draw the…
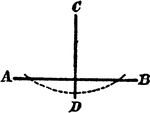
Construction Of A Perpendicular
An illustration showing the construction used to erect a perpendicular from a point to a line. "With…
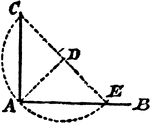
Construction Of A Perpendicular
An illustration showing the construction used to erect a perpendicular at the end of a line. "With the…
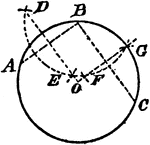
Find The Center Of A Circle Through 3 Points
An illustration showing how to find the center of a circle which will pass through three given points…
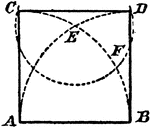
Square Constructed Upon A Given Line
An illustration showing how to construct a square upon a given line. "With AB as radius and A and B…
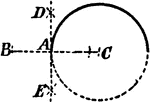
Construction Of Tangent To Circle
An illustration showing how to construct a tangent to a circle through a given point in a circumference.…
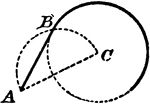
Construction Of Tangent To Circle
An illustration showing how to construct a tangent to a circle through a given point outside of a circumference.…
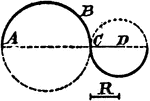
Construction Of Circle Tangent To Circle
An illustration showing how to construct a tangent circle to a circle with a given radius. "Through…
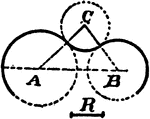
Construction Of Circle Tangent To 2 Circles
An illustration showing how to construct a tangent circle to 2 given circles. "Join centers C and c…