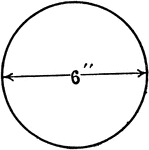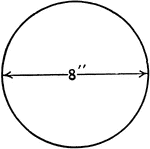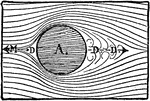
Circle Aerodynamic
A circle in a aerodynamic chamber where the airflow hitting the object. The area at point DD is large…
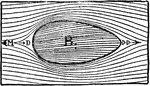
Ellipse Aerodynamic
Aerodynamic of the ellipse where the area at point DD is small. The small area creates less resistance…
Golden Angle
Illustration showing the golden angle. The golden angle is the smaller of two angles created by dividing…
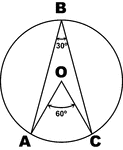
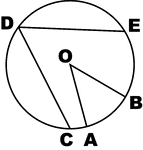
Inscribed and Central Angles 30 60
Illustration of circle with inscribed angle 30 and central angle 60.
Annulet
"Azure, an annulet argent. Annulets are added to arms for a difference. ANNULET. A small circle borne…
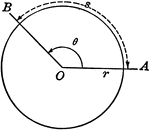
Model of Arc Length, Angle Measure, and Radius
Illustration showing that the arc length can be found by multiplying the angle measure by the radius…
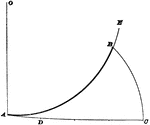
Construction Of Arc
Illustration used to show how to "find an arc of a circle having a known radius, which shall be equal…
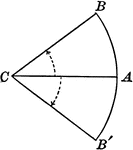
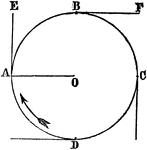
Positive and Negative Arcs in Circles
Illustration showing arcs measured in positive and negative angles.

Assemble, March
"Raise the arm vertically to its full extent and describe horizontal circles." — Moss, 1914
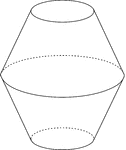
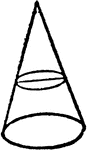
Conical Bifrustum
Illustration of a conical bifrustum created by three parallel planes of circles with the middle plane…
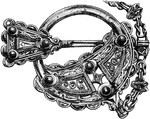
Round Brooch
This round brooch is in the shape of a circle with a half moon shape laying on the interior bottom edge…

Vertical Cross Section of Spherical Zones of a Casting
An illustration of a vertical cross section of the spherical zones of a casting with a diameter of 16…
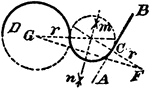
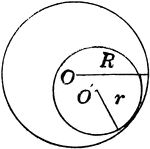
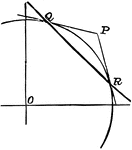
Construction Of A Center And Radius Of A Circle That Will Tangent A Given Circle And Line
An illustration showing how to construct the center and radius of a circle that will tangent a given…
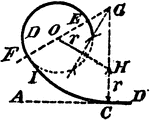
Construction Of A Center And Radius Of A Circle That Will Tangent A Given Circle And Line
An illustration showing how to construct the center and radius of a circle that will tangent a given…
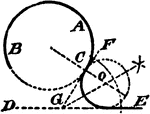
Construction Of A Center And Radius Of A Circle That Will Tangent A Given Circle
An illustration showing how to construct the center and radius of a circle that will tangent a given…
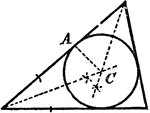
Construction Of The Center And Radius Of A Circle Tangent To Triangle Sides
An illustration showing how to construct a center and radius of a circle that will tangent the three…
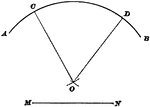
Construction Of Center
Illustration used to show how to find the center when given an arc and its radius.
Centrifugal Force
"Attach a ball, for instance, to a cord; and , fastening the end of the cord at a point, O, give a quick…
Centrifugal Force
"The instant one of the strings is let go, the centrifugal force carries off the stone in a tangent…
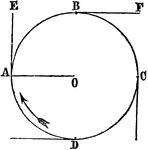
Circle
Illustration showing a circle formed by the intersection of a plane perpendicular to the axis of the…
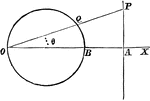
Construction Of A Circle Arc
An illustration showing how to construct a circle arc without recourse to its center, but its chord…
Construction of Circle Given 3 Points
Illustration used to construct a circle when given three points.

Marble Mosaic Circle Pattern
This marble mosaic circle pattern is inlaid pieces of glass found in the windows of a cathedral in Florence,…

Marble Mosaic Circle Pattern
The marble mosaic circle pattern is inlaid pieces of stone, wood, glass, leather or straw to make a…

Medieval Tile Circle Pattern
This Medieval Tile circle pattern is a stained glass design. It the oldest process of fitting together…

Medieval Tile Circle Pattern
This medieval tile circle pattern is a stained glass design. It the oldest process of fitting together…

Medieval Tile Circle Pattern
This medieval tile circle pattern is a stained glass design. It the oldest process of fitting together…
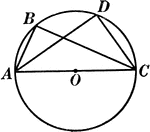
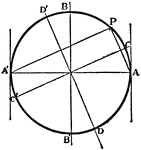
Angles Inscribed in the Same Segment Circle Proof
Illustration of a circle used to prove "All angles inscribed in the same segment are equal."
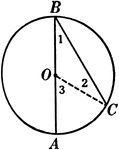
Inscribed Angle in a Circle Proof
Illustration of a circle with an inscribed angle that can be used to prove that "An inscribed angle…
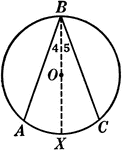
Inscribed Angle in a Circle Proof
Illustration of a circle with an inscribed angle that can be used to prove that "An inscribed angle…
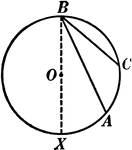
Inscribed Angle in a Circle Proof
Illustration of a circle with an inscribed angle that can be used to prove that "An inscribed angle…
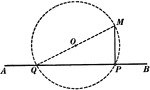
Obtuse Angles Inscribed in Circle Proof
Illustration of a circle used to prove "Any angle inscribed in a segment less than a semicircle is an…
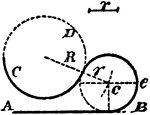
Construction Of A Circle Tangent To A Line And A Circle
An illustration showing how to construct a circle tangent to a given line and given circle. "Add the…
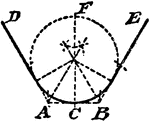
Construction Of A Circle That Tangents 2 Given Lines And Goes Through A Given Point
An illustration showing how to construct a circle that tangents two given lines and goes through a given…
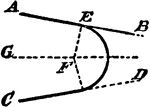
Construction Of A Circle That Tangents 2 Given Lines
An illustration showing how to construct a circle that tangents two given lines inclined to one another…
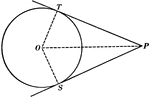
Equal Tangents to Circle Theorem
Illustration used to show that "If two tangents are drawn from any given point to a circle, those tangents…
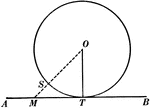
Tangent to Perpendicular Radius Circle Theorem
Illustration used to show that "A tangent to a circle is perpendicular to the radius drawn to the point…
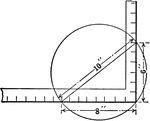
Circle With 10 inch Diameter and Steel Square
Illustration of circle with 10 inch diameter and square.
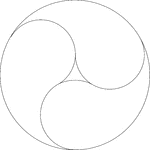
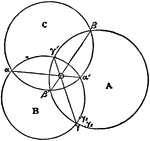
3 Yin Yang Design Symbols In A Circle
Design made by drawing one large circle and then three circles that are internally tangent to the original…

3 Yin Yang Design Symbols In A Circle
Design made by drawing one large circle and then three circles that are internally tangent to the original…
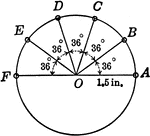
Circle With 36 degree Angles and Radius 1.5 in.
Circle with 36 degree angles marked. This diagram can be used with the following trig problem: Locate…
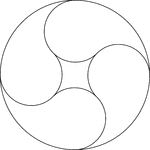
4 Yin Yang Design Symbols In A Circle
Design made by drawing one large circle and then four circles that are internally tangent to the original…
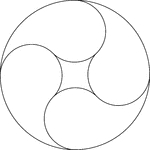
4 Yin Yang Design Symbols In A Circle
Design made by drawing one large circle and then four circles that are internally tangent to the original…

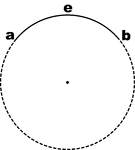
Arc of Circle
An illustration of an arc of a circle. An arc is any part of the circumference of a circle.
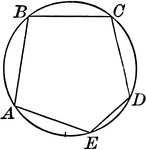
Circle with Arc, Chord, Inscribed Angle, Circumscribed Polygon
Illustrations of a circle with arc, chord, inscribed angle, and circumscribed about a polygon.