The Circles ClipArt gallery offers 166 Illustrations of circles with radii, diameters, chords, arcs, tangents, secants, and inscribed angles. Images also include inscribed, circumscribed, and concentric circles.
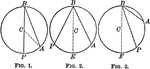
Circles With Inscribed Angles
Illustration of equal circles to show that an inscribed angle is measured by half the arc intercepted…
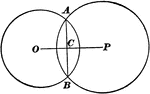
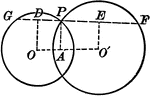
Intersecting Circles
Illustration of intersecting circles. "If two circles intersect each other, the line joining their centers…
Intersecting Lines in Circles
Illustration showing various circles and the angles formed by intersecting lines.
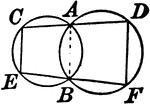
Two Intersecting Circles With Lines
Illustration showing 2 intersecting circles with a line drawn through each point of intersection terminated…
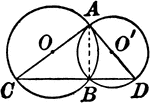
Two Intersecting Circles With Lines
Illustration showing 2 intersecting circles with a lines drawn that form a triangle.
Circles With Parallels Intercepting Equal Arcs
Illustration of a circle with parallels intercepting equal arcs on a circumference.
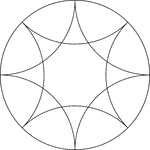
Reflected Arcs Of 2 Circles In A Circle
A design created by dividing a circle into 4 equal arcs and creating a reflection of each arc toward…
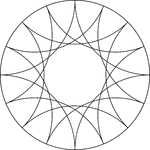
Reflected Arcs Of 4 Circles In A Circle
A design created by dividing a circle into 4 equal arcs and creating a reflection of each arc toward…
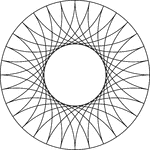
Reflected Arcs Of 8 Circles In A Circle
A design created by dividing a circle into 4 equal arcs and creating a reflection of each arc toward…
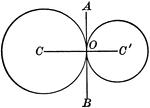
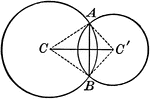
Tangent Circles
Illustration of two circles that are tangent to each other, the line of center passing through the point…
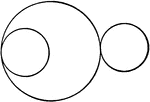
Tangent Circles
Illustration of tangent circles. One circle is said to be tangent to another circle when they touch…
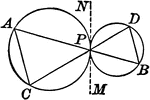
Tangent Circles With Chords
Illustration showing 2 circles with that touch each other and two lines drawn through the point of contact…
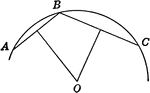
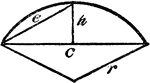
Construction of Arc When Given the Chord and Height of the Segment
Illustration of of construction of an arc when the chord and height of the segment are given.
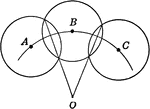
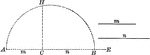
Construction of Radius When Given Only a Part of the Circumference
Illustration of of construction of a radius when given only a part of the circumference.
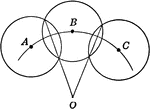
Construction of Radius When Given Only a Part of the Circumference
Illustration of of construction of a radius when given only a part of the circumference.

Design Similar to Yin Yang Symbol
Design made by drawing one large circle and then two circles that are vertically placed and internally…

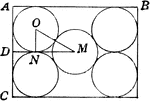
Circular Design
Circular design made by rotating circles about a fixed point. The radii of the smaller circles is equal…

Circular Design
Circular design made by rotating circles about a fixed point. The radii of the smaller circles is equal…
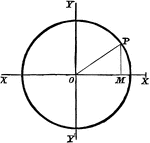
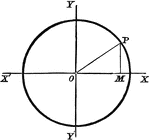
Circle Equation
To find the equation of a circle whose center is the origin and whose radius is 3 units in length.

Gyroscope
Three suspended concentric circles free to move independently of each other at right angles.
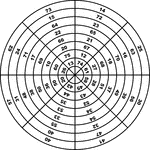
Magic Circle of Circles
The magic circle of circles, first developed by Benjamin Franklin, consists of eight annular rings and…
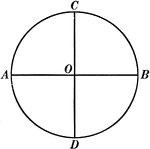
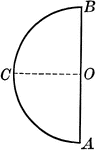
Circle With 2 Perpendicular Diameters
Illustration of a circle with center O and diameters AB and CD perpendicular to each other.
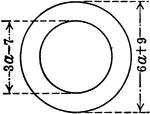
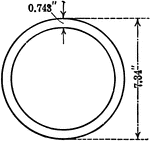
Ring Made of Circles With Diameters 3a-7 and 6a+9
An illustration of a ring (circle within circle), with diameters of 3a-7 and 6a+9. Illustration could…

Ring With Piece Cut Out
Illustration of ring (small circle in larger concentric circle) with piece cut out.
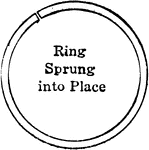
Ring Sprung Into Place
Illustration of ring (small circle in larger concentric circle) sprung into place.
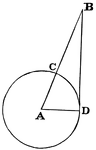
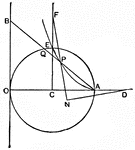
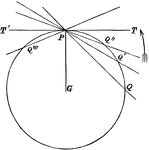
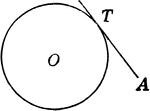
Circle with Secant
A secant is "a line which cuts a figure in any way. Specifically, in trigonometry, a line from the center…
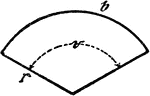
Circle Sector
An illustration showing a circle sector with radius r, center/central angle v, and length of circle…
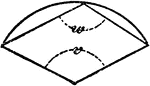
Circle Sector
An illustration showing a circle sector with center/central angle v and polygon angle w.

Signal Ahead, Silhouette
The Advance Traffic Control symbol signs includes the Signal Ahead sign. These signs shall be installed…
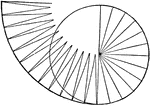
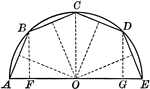
Circle Made Up Of Triangles
"A circle may be considered as made up of triangles whose bases form the circumference, and whose altitude…