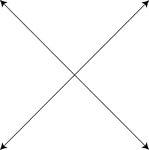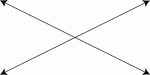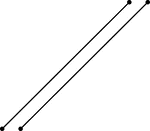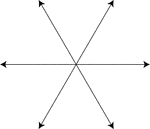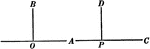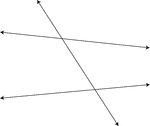
Transversal, 2 Lines Intersected By A
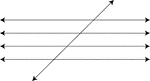
2 lines that are intersected by a third line known as a transversal.
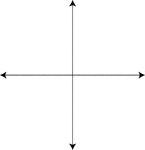
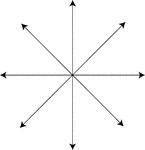
Intersecting Lines, 2 Perpendicular
2 intersecting lines, one of which is vertical and one of which is horizontal. The intersection forms…

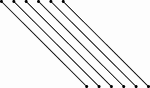
4 Parallel Lines Cut By 2 Transversals
Illustration used to prove the theorem "If three or more parallel lines intercept equal segments on…
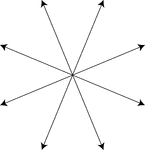
Segment, 3 Points on a Line
Illustration of line segment AB, which is made up of line segments AC and CB. C is considered the midpoint…
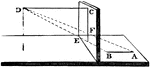
Accidental Point
"That point in which a right line drawn from the eye parallel to another given right line cuts the picture…
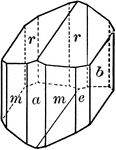
Albite
"Triclinic. Twinning very common, according to the albite law and evidenced by fine striation lines…
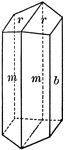
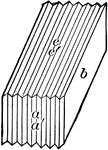
Amphibole
"Monoclinic. Crystals prismatic in habit; the prism faces make angles of 55 and 125 degrees with each…
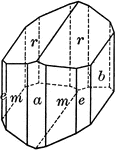
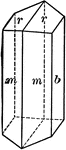
Amphibole
"Monoclinic. Crystals prismatic in habit; the prism faces make angles of 55 and 125 degrees with each…

Angelfish
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…
Angelfish
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…

Angelfish
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…
Angelfish
Tangrams, invented by the Chinese, are used to develop geometric thinking and spatial sense. Seven figures…

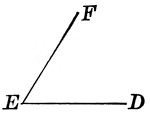
Acute Angle
An illustration showing an angle the is less than a right angle. This is an acute angle.
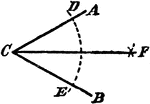
Construction Of A Divided Angle
An illustration showing the construction used to divide an angle into two equal parts. "With C as a…
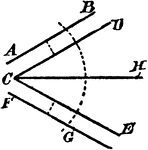
Construction Of A Divided Angle
An illustration showing the construction used to divide an angle into two equal parts when the lines…
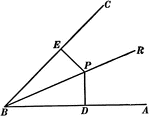
Perpendiculars To The Sides Of An Angle
Illustration used to show "The two perpendiculars to the sides of an angle from any point in its bisector…
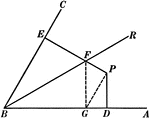
Perpendiculars To The Sides Of An Angle
Illustration used to show "The two perpendiculars to the sides of an angle from any point not in its…
Straight Angle
An illustration showing a straight angle. When the sides of an angle extend in opposite directions,…
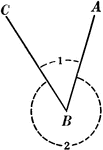
Acute and Reflex Angles
Illustration showing two positive angles; angle 1 being the acute angle and angle 2 being the reflex…
Acute, Obtuse, Straight, Right, And Reflex Angles
An illustration showing acute, obtuse, straight, right, and reflex angles.
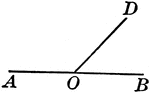
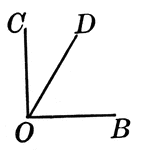
Adjacent Angles
An illustration of two angles that are adjacent. They have the same vertex and a common side between…
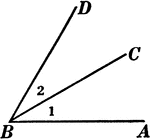
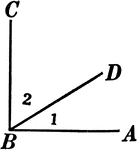
Adjacent Angles
Angles 1 and 2 are adjacent angles. Two angles with a common vertex and a common side between them are…
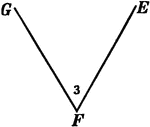
Difference of Angles
Illustration showing that the difference between angle 1 and angle 2 is angle ABC.
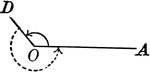
Obtuse And Reflex Angles
An illustration showing an angle the is greater than a right angle and less than a straight angle. This…